Molecular Dynamics Simulations: Elastic Characteristics of Sub-molecular Structural Elements
Cross-bridge stiffness. Coupling between the biochemical cycle and sarcomere mechanics is dictated by the strain dependence of the actomyosin cycle, which is itself dependent upon cross-bridge compliance. This compliance in turn resides in the S2, crossbridge “neck” region (stretching) and lever arm (bending). The most recent experiments report values of myosin stiffness from 1.5 to 3.2 pN/nm, which are much larger than most previous estimates used in sliding filament models. The higher estimates of crossbridge stiffness limit thermally-induced motions on unattached myosin heads and narrows parabolic parts of energy landscapes. This in turn increases the energy barriers between actomyosin states and reduces the probability of strain dependent transudation between them. Considering these basic uncertainties of mechanochemistry of actomyosin cycle, it is apparent that independent estimates of the cross-bridge stiffness from a study of its parts (S2, the lever arm, the “neck region”). To achieve this, we estimated elastic characteristics of crossbridge components from their known atomic structures by employing molecular dynamic simulations (CHARMM), using three different methods.
Elasticity of lever arm. The estimate of stretching stiffness of S2 suggests that very little crossbridge compliance resides in S2. Thus, likely the principal crossbridge compliance resides in bending of lever arm and eventual unfolding of the neck region. The lower bound of the lever arm lateral stiffness, estimated from the normal mode analysis of a-helix of the chicken myosin II lever arm (PDB code: 2MYS), is about 1.78 pN/nm (for ~10.58 nm long lever arm) which is about 1.5 times larger than the lateral stiffness for 10 nm long the poly-Ala a-helix (~ 1.2 pN/nm). Inclusion of side chains somewhat further increased the lever arm stiffness by a factor 2-3, i.e. cross-bridge lateral stiffness is in the range of 3.8 to 5 pN/nm. Accounting for the S2 stiffness and bending of the lever arm, the overall crossbridge stiffness is of the order 3.6-4.7 pN/nm.
Elasticity of neck region. Progress in modeling the actomyosin cross-bridge stiffness has been hampered by our lack of understanding of the mechanical properties of the `neck region'' located around the S1/S2 junction at P841 (chicken). The amino acid sequence in this region broadly follows the classical heptad repeat of coiled coils, although polar amino acids frequently substitute for hydrophobic residues in the classical heptad motif, which would most likely lead to an unstable coiled coil. Current crystal structures of myosins lack the neck region. Moreover, the S2 domains with the neck region at the N-terminus have been crystallized (scallop myosin II, and b-cardiac myosin II, and have found the N-terminal end to be either disordered or unstable. Several studies have suggested that coiled-coil unwinding is essential for the function of myosin, although some controversy remains. Thus, the neck region may be a highly flexible partially unwound section of the S2 domain that exists either as a random coil or a folded a-helix. Both of these alternatives could generate the necessary compliance in the cross-bridge: the a-helix through lateral bending stiffness and the random coil stiffness (through entropic elasticity) of ~ 2.75 pN/nm for a 100-residue random coil, assuming a persistence length of 1 nm, and low-force regime of the Worm-like Chain model for polymers.
Elasticity of S2. The stretching stiffness obtained by three methods from X-ray structure of S2 subdomain from scallop, and human was in range of 60 - 80 pN/nm (scaled to the 60nm long S2). This stiffness corresponds to apparent modulus (EA, where E is elastic modulus and A is cross-sectional area) of 3600-4800 pN and are consistent with stiffnesses estimated from S2 persistence lengths (of 100-200 nm).
Lateral and torsional stiffness of S2. The estimated flexural rigidity and cantilever lateral stiffness of human beta S2 and the scallop S2 are summarized in Table 1. It is interesting that flexural rigidity of human S2 is more than three times stiffer than the scallop S2 caused by a difference in only a few amino acids. The estimated torsional rigidity of the human S2 is 2610 pNnm2 is similar to that of S2 flexural rigidity.
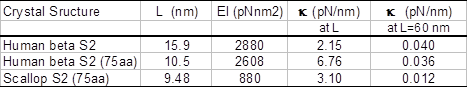
Table 1: Flexural rigidity and lateral stiffness of S2. L is length of the S2 sub-fragment with known crystal structure, EI is flexural rigidity, k is lateral stiffness (cantilever stiffness L nm long).
Unfolding of S2. We also estimated the cross-bridge force that can unfold S2. We found that unfolding of in S2 in axial direction is at forces > 30 pN, which is consistent with the unfolding force measurements by force atomic microscopy. In contrast the unfolding of coiled coils by applying a couple of forces perpendicular to axis of S2 (pulling myosin heads apart) occurred at much smaller force of ~10 pN.
Symmetry of energy landscapes. In all sliding filament models the elasticity of the cross bridge is assumed to be linear elastic. We showed above that stiffness of S2 is high and that S2 contributes very little to overall crossbridge compliance, at least in tension. Using estimated axial and lateral stiffnesses of S2 (of 60 pN/nm, and 0.01 pN/nm respectively), and bending stiffness S1 of 3 pN/nm, we computed force displacement relationship of a crossbridge in both tension and in compression. As expected, stiffness in tension was only slightly below 3p N/nm, whereas the stiffness falls about three times at compressive forces of 1 pN and falls more than an order of magnitude for forces larger than 3-4 pN. Consequently, the energy landscape is asymmetric and skewed toward negative crossbridge strains. More detailed analysis of the crossbridge stiffness in context of 3D sarcomere lattice is shown here.
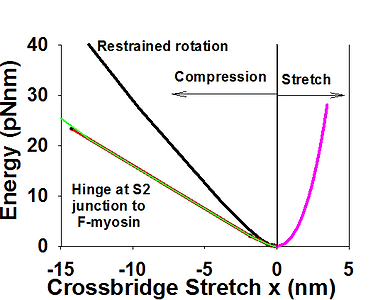
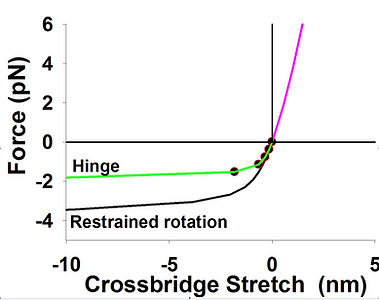
Asymmetric energy landscape in compression and tension (left) and force-stretch (right) calculated from FE analysis of a crossbridge in 3D sacomere lattice. Axial and lateral stiffness of S2 are 60 pN/nm and 0.01 pN/nm respectively, and lateral stiffness in lever arm of 3 pN/nm. Overall crossbridge stiffness drops rapidly in compression from 3 pN/nm at x=0 to ~0.5 at x=-2 nm. Imposing a hinge at the joint of S2 and myosin filament further reduces compliance of a crossbridge to compressive loads.
